Chapter 9. "Of Cubes, and of the Extraction of Cube Roots."
Part I. Section II. Chapter 9. “Of Cubes, and of the Extraction of Cube Roots.”
333 To find the cube of \(a+b\), we have only to multiply its square, \(a^2+2ab+b^2\), again by \(a+b\), thus;
| \(a\) | \(+2ab\) | \(+b^2\) | |
| \(a\) | \(+b\) | ||
| \(a^3\) |
\(+2a^2b\) | \(+ab^2\) | |
| \(a^2b\) | \(+2ab^2\) | \(+b^3\) | |
| \(a^3\) | \(+3a^2b\) | \(+3ab^2\) | \(+b^3\) |
We see therefore that it contains the cubes of the two parts of the root, and, beside that, \(3a^2b+3ab^2\); which quantity is equal to \((3ab)\cdot (a+b)\); that is, the triple product of the two parts, \(a\) and \(b\), multiplied by their sum.
334 So that whenever a root is composed of two terms, it is easy to find its cube by this rule: for example, the number 5 = 3 + 2; its cube is therefore 27 + 8 + (18 · 5) = 125.
And if 7 + 3 = 10 be the root; then the cube will be 343 + 27 + (63 · 10) = 1000.
To find the cube of 36, let us suppose the root 36 = 30 + 6, and we have for the cube required, 27000 + 216 + (540 · 36) = 46656.
335 But if, on the other hand, the cube be given, namely, \(a^3+3a^2b+3ab^2+b^3\), and it be required to find its root, we must premise the following remarks:
First, when the cube is arranged according to the powers of one letter, we easily know by the leading term \(a^3\), the first term \(a\) of the root, since the cube of it is \(a^3\); if, therefore, we subtract that cube from the cube proposed, we obtain the remainder, \(3a^2b + 3ab^2 + b^3\), which must furnish the second term of the root.
336 But as we already know, from Art. 333, that the second term is \(+b\), we have principally to discover how it may be derived from the above remainder. Now, that remainder may be expressed by two factors, thus, \((3a^2+3ab+b^2) \cdot (b)\); if, therefore, we divide by \(3a^2+3ab+b^2\), we obtain the second part of the root \(+b\), which is required.
337 But as this second term is supposed to be unknown, the divisor also is unknown; nevertheless we have the first term of that divisor, which is sufficient: for it is \(3a^2\), that is, thrice the square of the first term already found; and by means of this, it is not difficult to find also the other part, \(b\), and then to complete the divisor before we perform the division; for this purpose, it will be necessary to join to \(3a^2\) thrice the product of the two terms, or \(3ab\), and \(b^2\), or the square of the second term of the root.
338 Let us apply what we have said to two examples of other given cubes.
| \(a^3\) | \(+12a^2\) | \(+48a\) | \(+64\) | \(a+4\) | |
| \(3a^2+12a+16\) | \(a^3\) | ||||
| \(12a^2\) | \(+48a\) | \(+64\) | |||
| \(12a^2\) | \(+48a\) | \(+64\) | |||
| 0 |
| \(a^6\) | \(-6a^5\) | \(+15a^4\) | \(-20a^3\) | \(+15a^2\) | \(-6a\) | \(+1\) | \(a^2-2a+1\) | |
| \(a^6\) | ||||||||
| \(3a^4-6a^3-4a^2\) | \(-6a^5\) | \(+15a^4\) | \(-20a^3\) | |||||
| \(-6a^5\) | \(+12a^4\) | \(-8a^3\) | ||||||
| \(3a^4-12a^3+12a^2+3a^2-6a+1\) | \(3a^4\) | \(-12a^3\) | \(+15a^2\) | \(-6a\) | \(+1\) | |||
| \(3a^4\) | \(-12a^3\) | \(+15a^2\) | \(-6a\) | \(+1\) | ||||
| \(0\) |
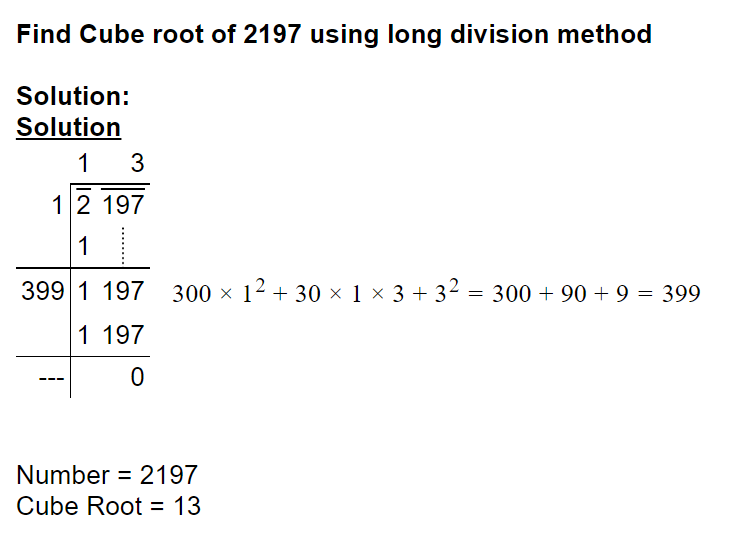
339 The analysis which we have given is the foundation of the common rule for the extraction of the cube root in numbers. See the following example of the operation in the number 2197:1 2
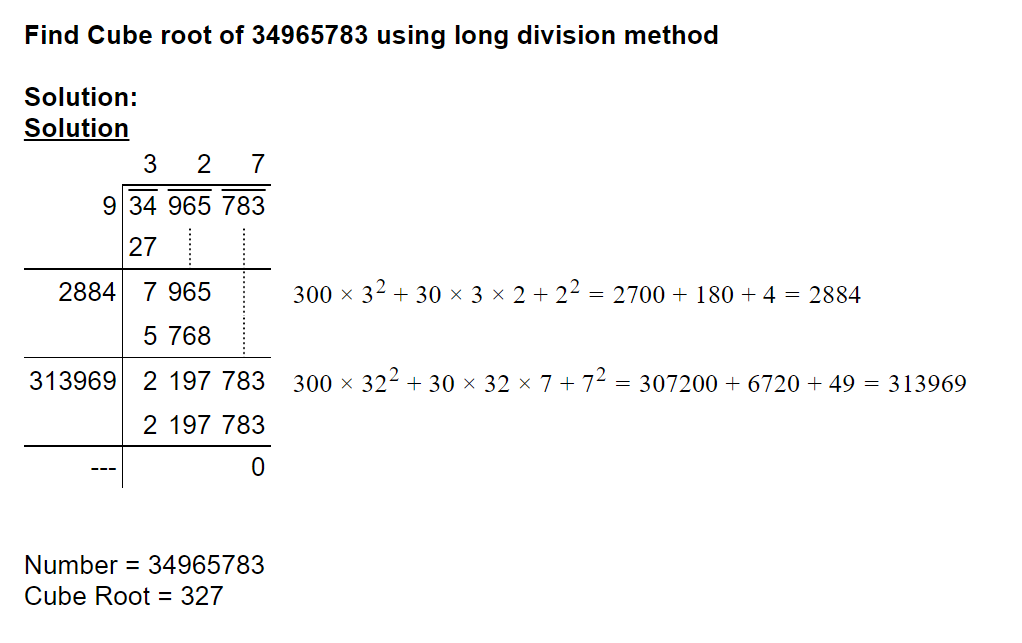
Let us also extract the cube root of 34965783:
Editions
- Leonhard Euler. Elements of Algebra. Translated by Rev. John Hewlett. Third Edition. Longmans, Hurst, Rees, Orme, and Co. London. 1822.
- Leonhard Euler. Vollständige Anleitung zur Algebra. Mit den Zusätzen von Joseph Louis Lagrange. Herausgegeben von Heinrich Weber. B. G. Teubner. Leipzig and Berlin. 1911. Leonhardi Euleri Opera omnia. Series prima. Opera mathematica. Volumen primum.
-
AtoZmath.com: Pre-Algebra Calculators. 12.2 Radicals. 8. Cube root of a number using long division method. ↩
-
Paul E. Black, “cube root”, in Dictionary of Algorithms and Data Structures [online], Paul E. Black, ed. 6 May 2019. Available from: https://www.nist.gov/dads/HTML/cubeRoot.html ↩